Definition of z score graph
The Z score graph depicts where the z score values are distributed. The horizontal axis of the Standard Normal Distribution is known as the Z-axis, and the values along it are known as Z-scores.
The z-axis is parallel to the x- and y-axes and is used to graph the value of z, mathematics’ third unknown. Positive Z-scores are plotted to the right of the mean, while negative Z-scores are plotted to the left.
A z-score graph depicts the position of a raw score in aspects of its standard deviation distance from the mean. The z-score in the z-score graph is positive if the value is greater than the mean and negative if it is less than the mean.
Finding area left of the z score
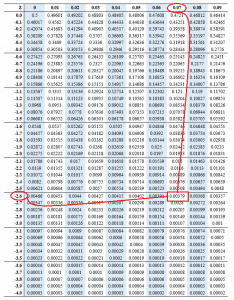
We can find the area left of the z score using a z table. The area left of the z score covers the region -z score table to 0. Notably, you have to understand what the percentages in the table mean. For instance, consider a z score of -1.1. We can find the area left of the z score by checking the z score table. Thus, we have;
The value of a -1.1 z score is 0.13567
This represents 13.57% of the area left of the z score. Similarly, a value of 0.49601 represents 49.601% of the area under a curve.
Examples of finding the area left of the z score
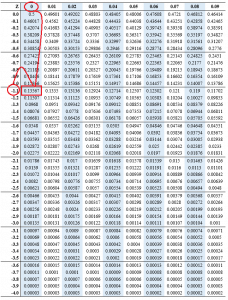
Example 2: Find the area left of the z score for value -0.38
The value of -0.38 z score is 0.35197
This represents 35.197% of the area left of the z score on the curve
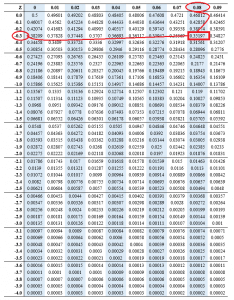
Example 3: Find the area left of the z score for value -2.67
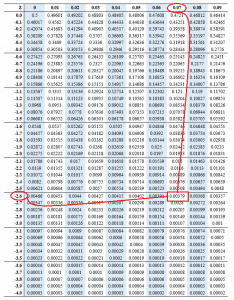
The value of a -2.67 z score is 0.00379
This represents 0.379% of the area left of the z score on the curve
find the z score for which the area to the left is 0.37
The z score for which the area to the left is 0.37 is -0.335
= (-0.33+-0.34)/2
=-0.335
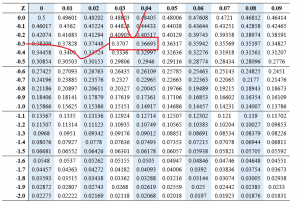
find the z score for which the area to the left is 0.22
The z score for which the area to the left is 0.22 is -0.77
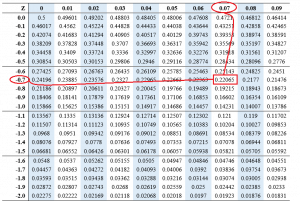
find the z score for which the area to the left is 0.96
The z score for which the area to the left is 0.96 is 1.75
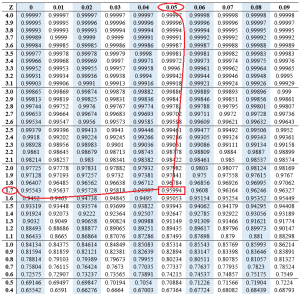
find the z score for which the area to the left is 0.79
The z score for which the area to the left is 0.79 is 0.81
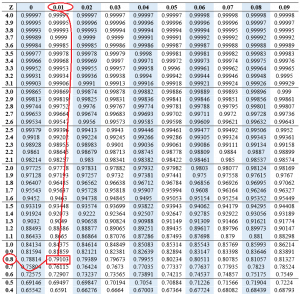
Finding the area right of the z score
To find the area to the right of the z score, we focus on the positive values of the standard normal distribution table. The area right of the z score is the area between the mean and z score table 0 to the z score table. To find the area right of the z score, we look up the value of z score and subtract it from 1.
Examples of finding area right of the z score
This example expounds on how you can find the area right of the z score.
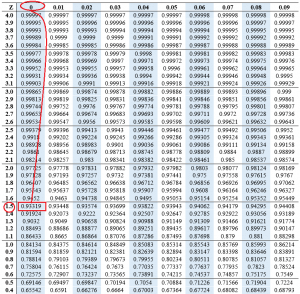
Example 1: Find area right of the 1.5 z score
The area right of the 1.5 z score is;
=1-0.93319
=0.06681
=6.681%
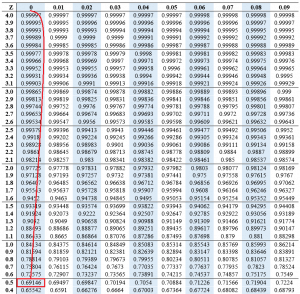
Example 2: Find area right of the 0.5 z score
The area right of the 0.5 z score is;
=1-0.69146
=0.30854
=30.854%
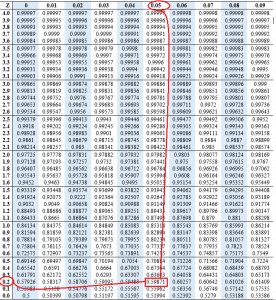
Example 3: Find the area right of the 0.25 z-score
The area right of the 0.25 z score is;
=1-0.59871
=0.40129
=40.129%
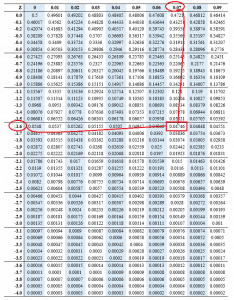
Example 4: Find the area right of the -1.67 z-score
The area right of the -1.67 z-score is;
=1-0.04746
=0.95254
=95.254%
Example 5: Find area right of the -2.67 z-score
The area right of the -2.67 z-score is;
=1-0.00379
=0.99621
=99.621%