To calculate confidence interval for a z score, we use the formula;

To calculate confidence interval for a z score, we follow the following steps;
For example, let’s say the sample size is 64, the sample mean is 5, and the standard deviation of 0.8. Calculate the confidence interval of 95% for the z score.
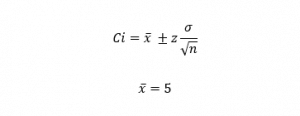
Z score of 95% confidence interval is 1.96

Example 2: A random sample of 100 students has an average mean of 3.5. Assuming that the standard deviation is 0.64. Construct a 97% confidence interval for the population mean.
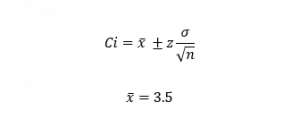
Z score of 97% confidence interval is 2.17

Example 3: In a manufacturing company, the raw materials’ weight is normally distributed with a mean of 15kgs and a standard deviation of 2 kgs. Construct a 92% confidence interval for 121 products made from raw materials.
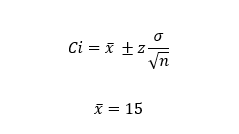
Z score of 92% confidence interval is 1.75
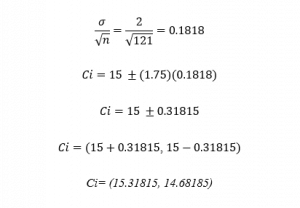
What is significance level and how it relates to confidence interval
The significance level is the probability that an event happened by chance and determines the relevance of the event. It measures how much evidence is needed in the dataset before rejecting the null hypothesis and concluding that the effect is statistically significant.
A significance level of 0.02, for example, indicates that there is a 2% possibility of detecting a differentiation when none exists. Lower degrees of significance implies that more evidence is required to reject the null hypothesis.
To be declared statistically significant, the sample mean must be separated from the null hypothesis by a certain amount. The confidence level specifies the distance between the sample mean and the confidence boundaries.
Examples of significance level
There are various examples of the significance level. This value is chosen considering factors such as the estimated size, the sample size, and the consequences that follow a given risk level.
The common examples of significance levels are 0.05 (which is one chance in 20), 0.01 level of significance, and 0.10 level of significance.
0.01 level of significance z score
A significance level of 0.01 indicates a 1% risk of detecting a difference that exists. This refers to 1 chance in 100.
1 per cent level of significance z score
1% level of significance is the same as 0.01 level of significance z score, which refers to 1 chance in 100.
When is a z score unusual?
An unusual Z-score is one that is less than -1.96 and larger than 1.96 in general. These z-score values are referred to as exceptional because the interval (-1.96,1.96) covers 95 percent of the area. Only 5% of z-score values go outside of this range. As a result, any Z score of more than 2 (in absolute value) is considered exceptional.
