What is Z score normalization?
Z-score normalization is the process of transforming each item in a dataset so that their mean is 0 and their standard deviation is 1.
Z score normalization formula
The Z score normalization formula is;
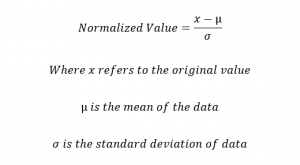
Difference between Z score and Z score Normalization
Z score represents the number of standard deviations from the mean the data points differ. In contrast, Z score Normalization or Standardization refers to the process of converting raw data or observation into a Z score.
Z score normalization example
Normalize the following data points from the following dataset.
4,6,7,9,10,13,15,14,16,17,18,18,20,8,5
Step 1: Calculate the Mean
We commence by calculating the mean

The mean is 15.2667
Step 2: Calculate the Standard deviation
The standard deviation is given by;
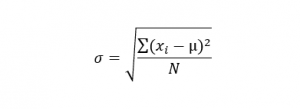
The value of the standard deviation is 11.3986
Step 3: Normalizing the data points
After getting the mean and the standard deviation, we now normalize the data points using our formula. The Normalized value of the first value will be;
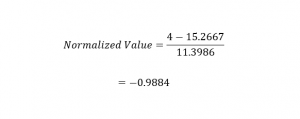
The other normalized data values will be;
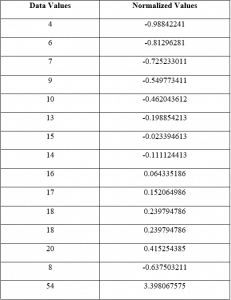
From this data normalization, we can clear the outliers in the dataset. For example, in our data, 54 is an outlier and has been normalized so that it is no longer a big outlier. This makes the outlier fit in a model, and it will not significantly influence the molding of the model.
